A spiral is made up of successive semicircles, with centres alternately at A and B, starting with centre at A, of radii 0.5 cm, 1.0 cm, 1.5 cm, 2.0 cm, . . . as shown in Fig. 5.4. What is the total length of such a spiral made up of thirteen consecutive semicircles? (Take π = 22/)
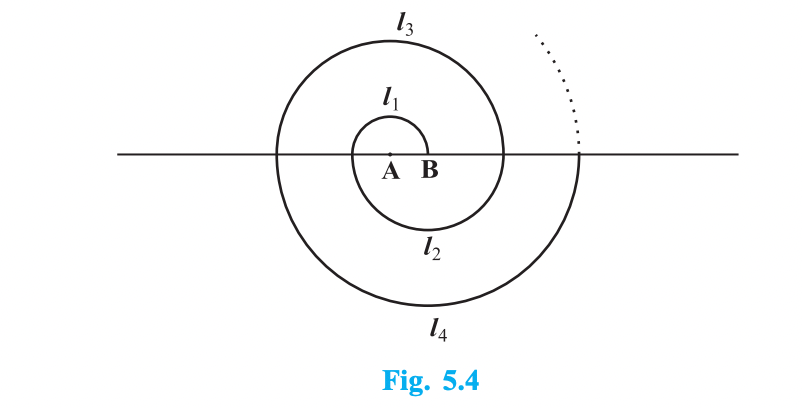
[Hint: Length of successive semicircles is l1, l2, l3, l4, . . . with centres at A, B, A, B, . . ., respectively.]
Solution:
We know,
The perimeter of a half-circle shape (as shown in the figure) = π r
Therefore,
l1 = π(0.5) = π/2 cm
l2 = π(1) = π cm
l3 = π(1.5) = 3π/2 cm
Where, l1, l2, and l3 are the lengths of the half-circle shapes.
Hence we have a series here, as,
π/2, π, 3π/2, 2π, ….
l1 = π/2 cm
l2 = π cm
Common difference, d = l2 – l1 = π – π/2 = π/2
First term = l1= a = π/2 cm
By the sum of the n terms formula, we know,
Sn = n /2 [2 a + ( n – 1) d ]
Therefore, the sum of the length of 13 consecutive circles is;
S13 = 13 /2 [2(π/2) + (13 – 1)π/2]
= 13 /2 [π + 6π]
=13 /2 (7π)
= 13 /2 × 7 × 22 /7
= 143 cm