Solution:
Given equations are x – y + 1 = 0 and 3x + 2y – 12 = 0.
Let’s consider the equation x – y + 1 = 0.
y = x + 1
| x | 0 | 1 | 2 |
| y | 1 | 2 | 3 |
Now, consider the second equation 3x + 2y – 12 = 0
2y = 12 – 3x
y = (12 – 3x)/2
| x | 0 | 2 | 4 |
| y | 6 | 3 | 0 |
Hence, the graphical representation of these equations is as follows.
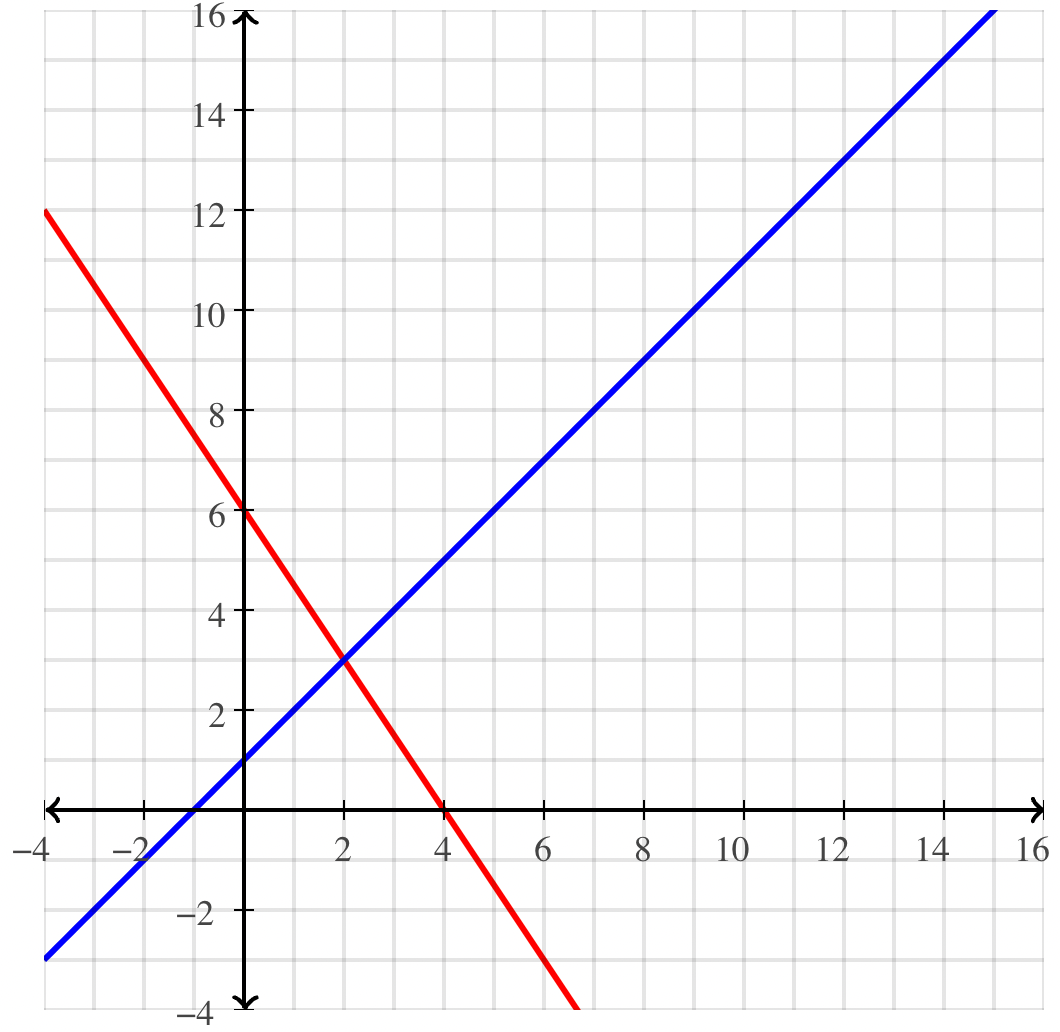
From the figure, it can be seen that these lines are intersecting each other at points (2, 3) and the x-axis at (−1, 0) and (4, 0).
Therefore, the vertices of the triangle are (2, 3), (−1, 0), and (4, 0).